Advanced Speed Distance and Time short tricks for competitive exams are discussed here. Quantitative aptitude questions related to speed, distance, and time are frequently asked in various competitive exams and job interviews. To solve these problems, it is important to understand the basic formulae and concepts related to speed, distance, and time.
One must also be able to convert between different units of measurement, such as kilometers per hour (km/hr) and meters per second (m/s). Practice and familiarity with common types of problems, such as those involving relative speed, average speed, and round-trip journeys, can also help improve one’s ability to solve speed, distance, and time problems quickly and accurately.
Basic Formula
Let a person running with a speed of ‘s’ cover a distance from A to B in time ‘t’. Here AB = d.
Then, Distance= Speed×Time
d=s×t
Proportionality between speed, distance, and time
Here we will explore the relationship between speed, distance, and time in three different scenarios.
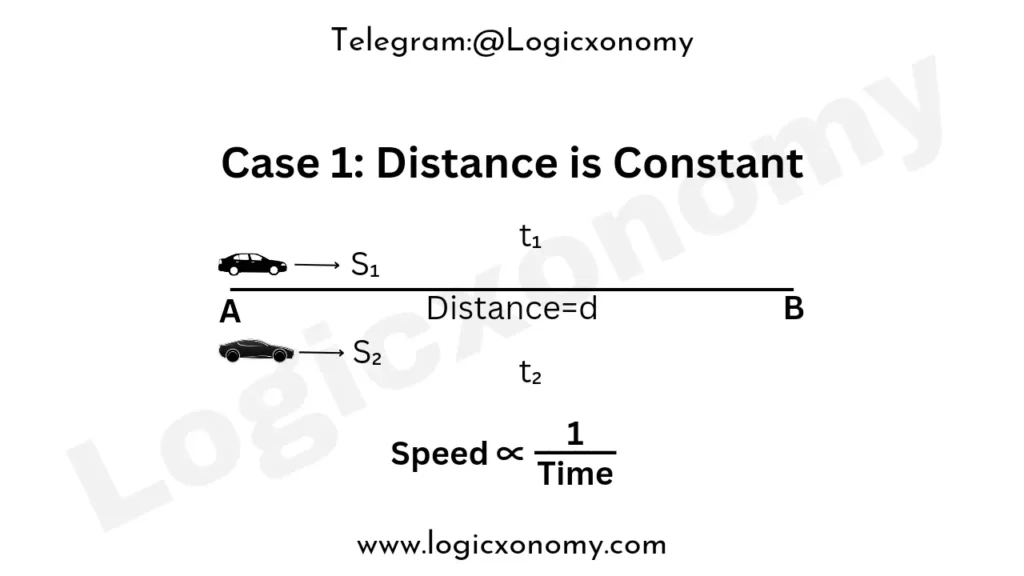
Case 1: Distance is constant
Let two persons traveling with speeds s1 and s2 cover the same distance (d) in time t1 and t2 respectively.
Here we will use the standard formula for both persons.
Distance= Speed×Time
d=s1×t1 ………(i)
d=s2×t2 ………(ii)
Compare both equations:
s_1\times t_1=s_2\times t_2.
\frac{s_1}{s_2}=\frac{t_2}{t_1}.
Speed ∝\frac{1}{Time}.
It means that if the distance is fixed then speed is inversely proportional to time.
t_1=\frac{d}{s_1} and t_2=\frac{d}{s_2}.
Time difference (△t)=|t1-t2|
Speeds difference (△s)=|s1-s2|
\triangle t=d\times (\frac{1}{s_1}-\frac{1}{s_2}).
\triangle t=d\times (\frac{s_2-s_1}{s_1\times s_2}).
d=(\frac{s_1\times s_2}{\triangle s})\times\triangle t.
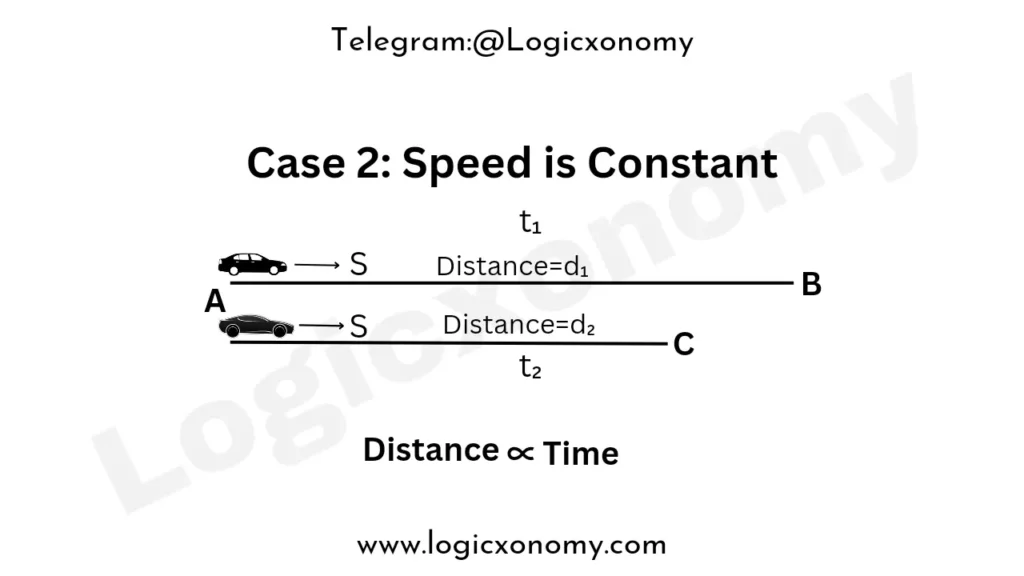
Case 2: Speed is constant
Let two persons traveling at the same speed ‘s’ cover a distance d1 in time t1 and distance d2 in time t2 respectively.
Distance= Speed×Time
d1=s×t1 ………(i)
d2=s×t2 ………(ii)
Divide equation (i) by (ii):
\frac{d_1}{d_2}=\frac{t_1}{t_2}.
Distance ∝ Time
It means that if the speed is constant, then the distance is proportional to the time.
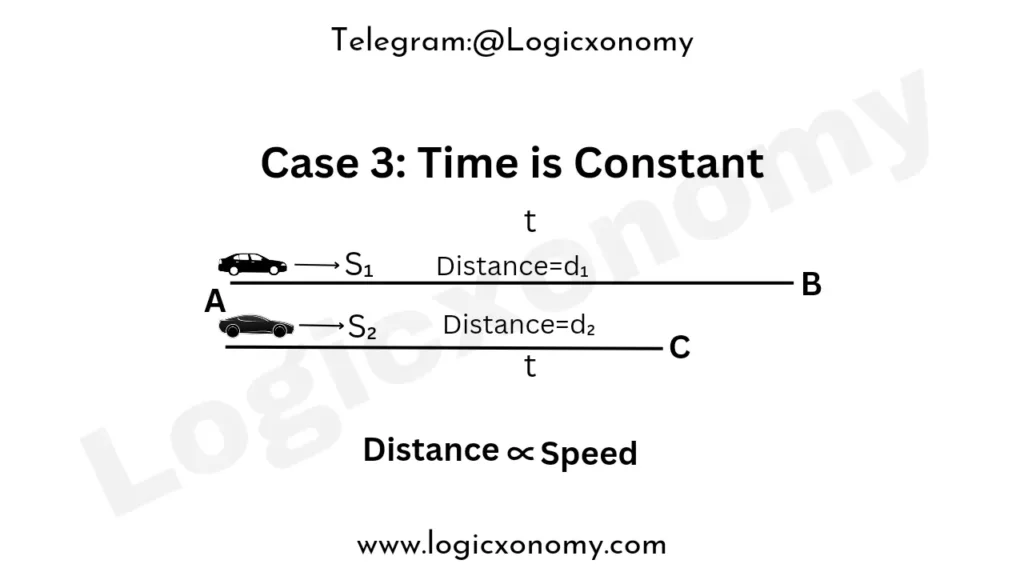
Case 3: Time is constant
Let two persons traveling with speeds s1 and s2 cover a distance d1 and distance d2 respectively in the same time t.
Distance= Speed×Time
d1=s1×t ………(i)
d2=s2×t ………(ii)
Divide equation (i) by (ii):
\frac{d_1}{d_2}=\frac{s_1}{s_2}.
Distance ∝ Speed
It means that if the time is constant, then the distance is proportional to the speed.

Speed Distance and Time Short Tricks
Que 1: Ramesh reaches his office 16 minutes late at three fourth of his normal speed. Find the normal time taken by him to cover the distance between his home and his office.
Method 1: △t= 16 min and △s= s/4
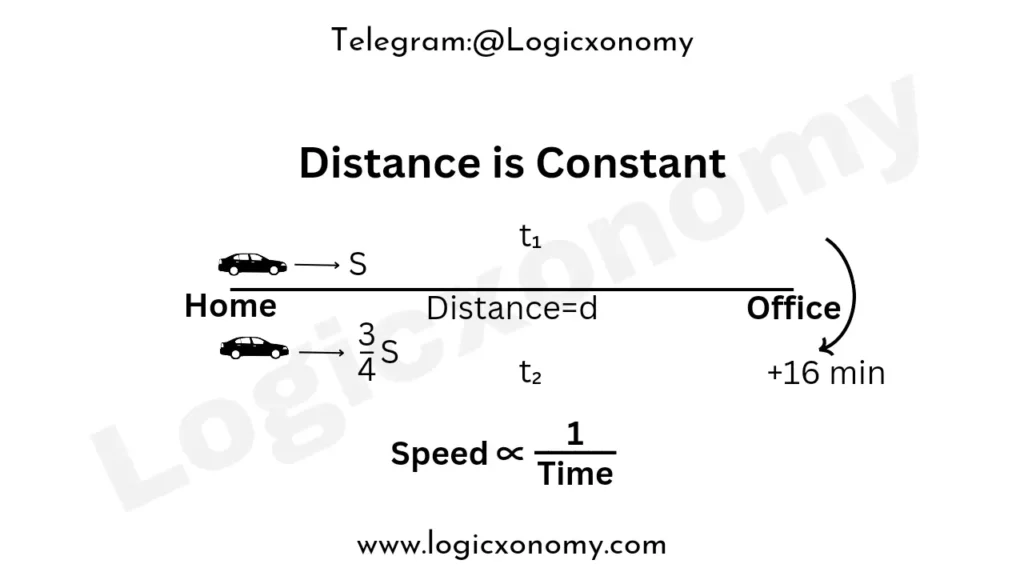
d=(\frac{s_1\times s_2}{\triangle s})\times\triangle t.
d=(\frac{\frac{3}{4}s^2}{\frac{s}{4}})\times 16= 48s.
\frac{d}{s}=48.
t= 48 min
Method 2: Speed ∝\frac{1}{Time}.
Speed⇒ 1 : ¾ =4 : 3
Time⇒ 3 : 4
Time difference (△t)= 4-3=1 unit= 16 min
Normal time duration= 3 unit= 3×16=48 min
Que 2: Ram and Shyam cover the same distance at the speed of 6 km/hr and 10 km/hr respectively. If Ram takes 30 minutes more than Shyam, find the distance covered by each.
Solution: △t= 30 min= ½ hr and △s= 10-6= 4km/h
d=(\frac{s_1\times s_2}{\triangle s})\times\triangle t.
d=(\frac{10\times 6}{4})\times\frac{1}{2}.
Distance= 7.5 km
Que 3: If Rohan reduces his speed by 5 km/h, he will take four hours more to reach the destination. If he increases his speed by 5 km/h, he will take 2 hours less to reach the destination. Find the normal time taken by him.
Solution: In the first case, if Rohan reduces his speed by 5 km/h, he takes four hours more.
△t= 4 hr and △s= 5 km/h (Speed Distance and Time Short Tricks)
d=(\frac{s_1\times s_2}{\triangle s})\times\triangle t.
d=(\frac{x.(x-5)}{5})\times 4 ………..(i)
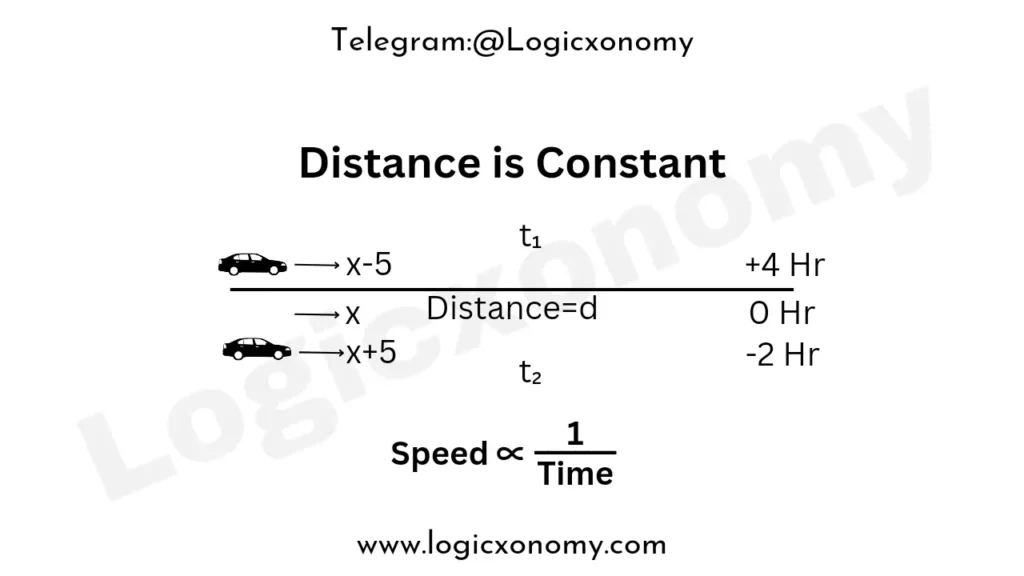
In the second case, if Rohan increases his speed by 5 km/h, he takes two hours less.
△t= 2 hr and △s= 5 km/h
d=(\frac{s_1\times s_2}{\triangle s})\times\triangle t.
d=(\frac{x.(x+5)}{5})\times 2 ………..(ii)
Now compare both equations:
(\frac{x.(x-5)}{5})\times 4=(\frac{x.(x+5)}{5})\times 2.
2\times (x-5)=x+5.
Normal Speed (x)= 15 km/h
Now put this value of x into any of the above equations:
Distance (d)=120 km
The normal time taken to complete the journey =d/x= 8 hr
Permutations and Combinations concepts and tricks: Click Here

