Here is the Proof of Routh’s Theorem to find the area of the triangle formed by pairwise intersections of three Cevians of the triangle.
Find the ratio of the Areas of ∆PQR & ∆ABC. If in the given triangle AD, BE & CF are the Cevians, drawn such that
Cevian: A line that intersects a triangle’s vertex and the side opposite that vertex.
\frac{CD}{BD}=x.
\frac{AE}{EC}=y.
\frac{BF}{FA}=z .
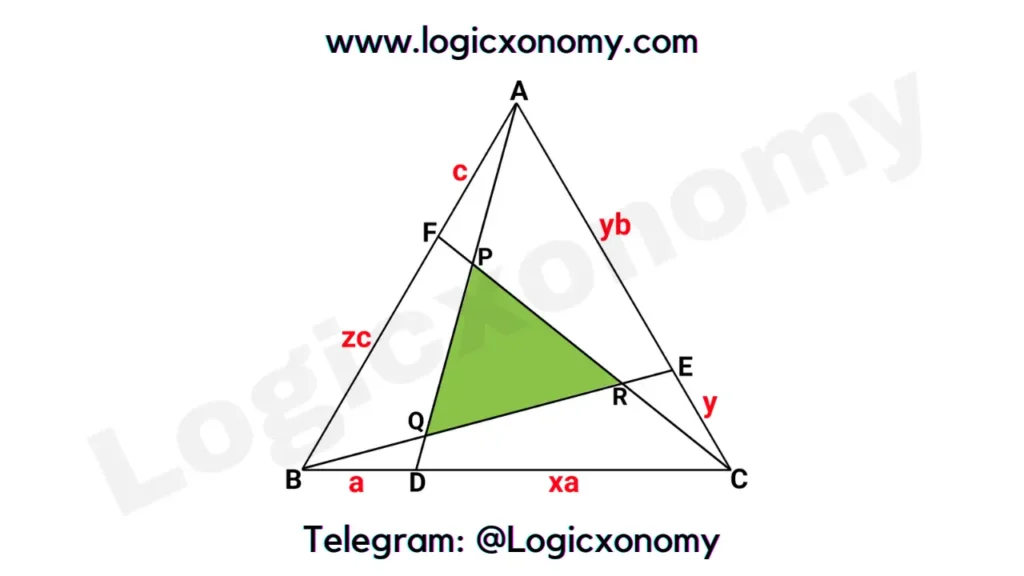
Step 1: Apply Menelaus Theorem in ∆BAD, (Click here for Menelaus Theorem)

\frac{BC}{DC}×\frac{DP}{PA}×\frac{AF}{FB}=1.
\frac{a(1+x)}{xa}×\frac{DP}{PA}×\frac{c}{zc}=1.
\frac{DP}{PA}=\frac{xz}{1+x}.
\frac{AP}{AD}=\frac{AP}{AP+PD}=\frac{1+x}{1+x+xz}.
Area of ∆APC/Area of ∆ADC=AP/AD
\frac{AP}{AD}=\frac{1+x}{1+x+xz}…….(i)
Area of ∆ABC/Area of ∆ADC=BC/DC
\frac{BC}{DC}=\frac{a(1+x)}{ax}=\frac{1+x}{x}……….(ii)
Equation (i)÷(ii)
Area of ∆APC/Area of ∆ABC=x/(1+x+xz)
Area of ∆APC=x∆/(1+x+xz)…………. (Area of ∆ABC=∆)
Similarly,
Area of ∆AQB=y∆/(1+y+xy)
Area of ∆BRC=z∆/(1+z+yz)
Now, ar(∆PQR) =ar(∆ABC) – ar(∆APC +∆AQB+∆BRC)
=∆*[1 – x/(1+x+xz) – y/(1+y+xy) – z/(1+z+yz)]
\frac{ar(∆PQR)}{ar(∆ABC)}=\frac{(xyz-1)^2}{(xy+y+1)(yz+z+1)(zx+x+1)}

Application of Routh’s Theorem
Que 1: If CD:BD=BF:FA=AE:EC=2:1
Then x=y=z=2

In this case,
\frac{ar(∆PQR)}{ar(∆ABC)}=\frac{(x^3-1)^2}{(x^2+x+1)^3}
Put x=2 in this equation
ar(∆ABC)=7×ar(∆PQR)
Que 2: In the above figure, if AF: FB= 4:7, BD: DC=2:3 & CE: EA= 1:5 then find how much percentage is the △PQR of the △ABC (by area)?
Solution: To find the values of x,y & z. We can take either clockwise data or anti-clockwise data.
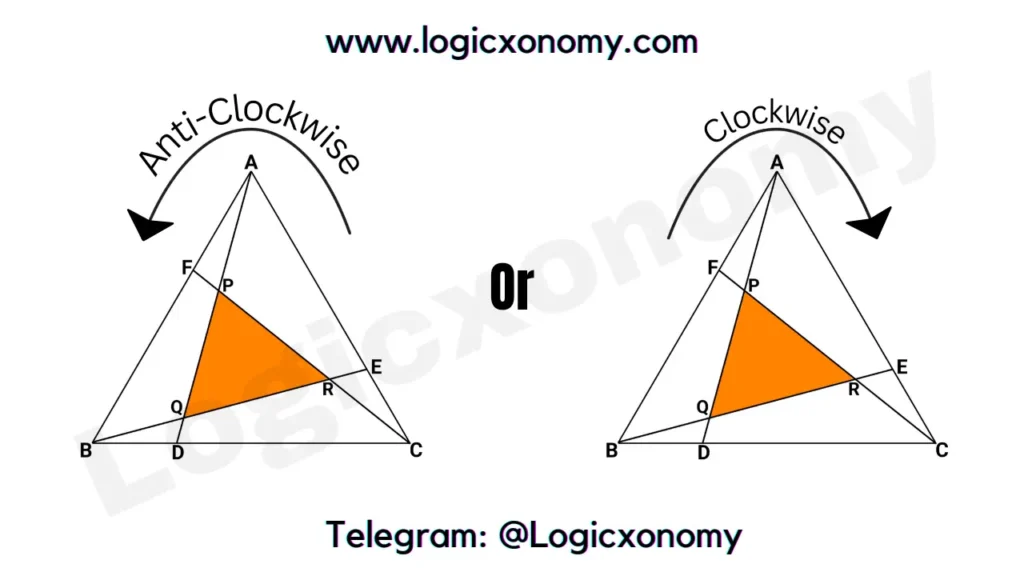
| Clockwise Data | Anti-Clockwise Data |
| AE: EC= 5:1= 5:1 CD: DB=3:2= \frac{3}{2}:1 FB: FA=7:4= \frac{7}{4}:1 Here x=5, y=\frac{3}{2}, z=\frac{7}{4}. | AF: FB= 4:7= 1:\frac{7}{4} BD: DC=2:3= 1: \frac{3}{2} CE: EA=1:5= 1:5 Here x=\frac{7}{4}, y=\frac{3}{2}, z=5. |
It means that in each case the answer will be the same.
The Routh’s Theorem:
\frac{ar(∆PQR)}{ar(∆ABC)}=\frac{(xyz-1)^2}{(xy+y+1)(yz+z+1)(zx+x+1)}.
Put, x=5, y=\frac{3}{2}, z=\frac{7}{4}.
\frac{ar(∆PQR)}{ar(∆ABC)}=\frac{97^{2}}{41\times 27\times 46}=\frac{9,409}{50,922}.
ar(△PQR)=18.5% of ar(△ABC) …….Approximately
Fast Calculation tricks (Mental Maths): Click Here

