Here we are going to discuss some very important Clock MCQ with solutions. This chapter comes under Logical Reasoning and is frequently asked in aptitude-based exams.

The hour hand completes two 360° rotations of the clock in a day and the minute hand completes one rotation in 1 hour while the Second hand completes one rotation in 1 minute.
1 Day= 24 Hour
1 Hour= 60 Minutes
1 Minute= 60 Seconds
So, In a day there are 24×60×60=86,400 Seconds. (Clock MCQ with solutions)
The circular dial of a clock is divided into 60 equal parts, called Minutes.
60 Minutes ⇒360°
1 Minute ⇒6°
In 1 hour, the hour hand covers 5 such parts which means the rotation of 5×6°=30°
So, the speed of Hour hand= \frac{30°}{60}= 0.5 Degrees/Minute
In 1 hour, the Minute hand covers 60 such parts which mean the rotation of 60×6°= 360°
So, the speed of the Minute hand= \frac{360°}{60}= 6 Degrees/Minute
The angle between Hour and Minute Hand
When we calculate the angle between the hour hand and the minute hand of a clock, two situations are possible
1. The minute hand is behind the hour hand
2. The minute hand is ahead of the hour hand
In the given figure, the angle between the baseline (0°) and the Minute hand is x° while the angle between the baseline and the Hour hand is y°.

Que: Find the angle between the hour hand and minute hand at H: M am or pm.
*(Here, H means hour and M is minute)
The rotation of Hour hand (y°)= Rotation in H hours + Rotation in M minutes
=Rotation in ‘60×H + M’ Minutes
=(60×H+M)×Speed
=(60×H+M)× 0.5 Degree/Minute
y=30×H+ 0.5×M ………..(i)
The rotation of Minute hand (x°)= Rotation in M minutes
=M×Speed
=M× 6 Degree/Minute
x=6×M ……….(ii)
Here in case:1 the angle between Hands (θ)= y-x
θ=(30×H+ 0.5×M)- 6×M
θ=30×H- 5.5×M
θ=30×H- \frac{11}{2}×M.
In case 2: the angle between Hands (θ)= x-y
θ=6M- (30×H+ 0.5×M)
θ=5.5×M-30×H
θ=\frac{11}{2}×M- 30×H.
So, the general formula of angle, θ=|30H-\frac{11}{2}M|.

Que 1: Find the angle between Hour and Minute hand at 10:15 AM.
Solution: θ=|30H-\frac{11}{2}M|.
Put, H=10, and M=15 (Clock MCQ with solutions)
θ=|30\times 10-\frac{11}{2}\times 15|.
θ=|300-82.5|.
θ=217.5° or 360°-217.5°=142.5°.
Que 2: Find the angle between Hour and Minute hand at 2:25 PM.
Solution: θ=|30H-\frac{11}{2}M|.
Put, H=2, and M=25 (Clock MCQ with solutions)
θ=|30\times 2-\frac{11}{2}\times 25|.
θ=|60-137.5|.
θ=77.5°.
Que 3: How many times in a day the angle between the hour and minute hands of a clock is 180 and 90 degrees?
Answer: The hands of the clock make 90 degrees twice per hour except at the time interval from 2 o’clock to 3 o’clock and 8 o’clock to 10 o’clock.
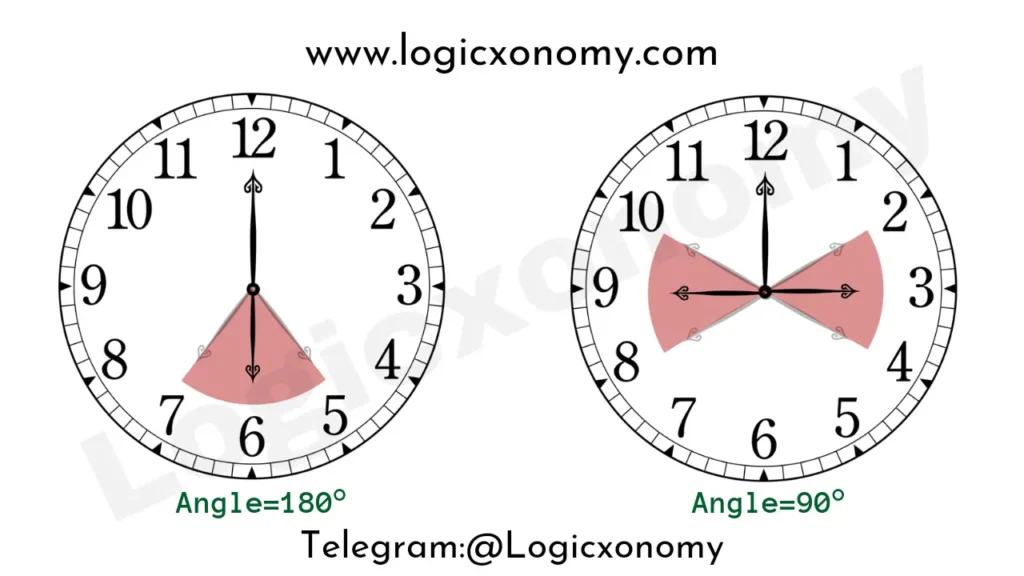
In the time interval from 2 o’clock to 3 o’clock and 8 o’clock to 10 o’clock, the hands of a clock make 90 degrees only 3 times instead of 4. This happens in both cycles of 12 hours each day.
So, the required result= 24×2- 4=44
The hands of the clock make 180 degrees once per hour, except from 5 o’clock to 7 o’clock. In this interval of 2 hours (5 to 7), an angle of 180 degrees is formed at 6 o’clock only. Means 11 times in 12 hours. (Clock MCQ with Solutions)
So, the required result= 24×1- 2=22
Note: The hour and minute hands of a clock make an angle of 90 degrees 44 times a day and 180 degrees 22 times a day.
Faulty Clock
A faulty clock either indicates more or less than real-time. If it indicates more, the clock is said to be a fast or time-gaining clock and if it indicates less, it is said to be a losing or slow clock.
Coinciding Time
We know that at 12 o’clock the hour hand and the minute hand coincide.
Now Find, after how many minutes both hands will meet again.
θ=|30H-\frac{11}{2}M|.
0=|30\times 12-\frac{11}{2}M|.
M=65\frac{5}{11} min
It means the hands of the clock coincide every 65\frac{5}{11} minutes.
Case (i): The coinciding time of a clock < 65\frac{5}{11} minutes
Fast or time-gaining clock
Case (ii): The coinciding time of a clock > 65\frac{5}{11} minutes
Slow or time-losing clock
Clock MCQ with Solutions
Que 1: Between 4 o’clock and 5 o’clock the hands of a clock make an angle of 90° twice, what is the interval between these two times?
(a) 32\frac{8}{11} min
(b) 28\frac{9}{11} min
(c) 24\frac{5}{11} min
(d) 28\frac{5}{11} min
Que 2: In a watch, the minute hand crosses the hour hand for the sixth time exactly after every 6 hrs 36 min 30 sec of watch time. What is the time gained or lost by this watch in one day? (Clock MCQ with Solutions)
(a) 14 min 10 sec lost
(b) 13 min 50 sec lost
(c) 13 min 20 sec gained
(d) 14 min 40 sec gained
Que 3: A clock that moves uniformly is 6 minutes slow at 8 am on Sunday and 6 minutes 48 seconds fast at 8 pm on the following Sunday. When was it right?
(a) 8:22:30 PM Wednesday
(b) 7:20:00 PM Wednesday
(c) 5:30:20 AM Thursday
(d) 7:20:00 AM Thursday
Que 4: A clock is set right at 5 AM. The clock loses 16 minutes in 24 hours. What will be the true time when the clock indicates 10 pm on the 4th day? (Clock MCQ with Solutions)
(a) 12:30 PM
(b) 12:00 PM
(c) 11:45 PM
(d) 11:00 PM
Que 5: How much does a clock gain/lose per day, if its hands meet every 64 minutes?
(a) 32 min gained
(b) 32\frac{8}{11} min lost
(c) 35\frac{5}{11} min gained
(d) 35 min lost
Que 6: The reflex angle between the hands of a clock at 10.25 is:
(a) 181.25°
(b) 192.5°
(c) 195°
(d) 197.5°
Que 7: A clock that gains 5 seconds in 3 minutes is correct at 7 in the morning. On the same day in the afternoon, when the clock strikes a quarter past four, the exact time is:
(a) 59\frac{7}{12} min past 3 pm
(b) 4 pm
(c) 58\frac{7}{11} min past 3 pm
(d) 2\frac{2}{11} min past 3 pm
Que 8: A clock that gains uniformly is 2 minutes slow on Monday at noon and 4 minutes 48 seconds fast on the next Monday at 2 pm. When was it right? (Clock MCQ with solutions)
(a) 2 pm on Tuesday
(b) 2 pm on Wednesday
(c) 3 pm on Thursday
(d) 1 pm on Friday
Que 9: There are two clocks, both set to show at 10 pm on 21st January 2010. One clock gains 2 minutes in an hour and the other clock loses 5 minutes in an hour. Then by how many minutes do the two clocks differ at 4 pm on 22nd January 2010?
(a) 126 min
(b) 136 min
(c) 96 min
(d) 106 min
Que 10: A wall clock gains 2 minutes every 12 hours, while a table clock loses 2 minutes every 36 hours. Both the watches are matched at 12 noon on Tuesday. When will the next time both show the same time?
(a) 12:30 at night after 130 days
(b) 12 noon after 135 days
(c) 12 midnight after 135 days
(d) 1:30 at night after 130 days
Que 11: In 25 min, the minute hand gains, over the hour hand by:
(a) 90°
(b) 122.5°
(c) 137.5°
(d) 150°
Que 12: A clock gains 15 min per day. It is set right at 12 noon. What time will it show at 4 am the next day? (Clock MCQ with solutions)
(a) 4:10 am
(b) 4:25 am
(c) 4:20 am
(d) 5:00 am
Que 13: If a clock strikes 12 in 33 seconds, it will strike 6 in how many seconds?
(a) 16.5 sec
(b) 15 sec
(c) 12 sec
(d) 30 sec
Que 14: At what time between 7 and 8 O’clock will the hands of a clock be in the same straight line but not together? (Clock MCQ with solutions)
(a) 5 min past 7
(b) 5(2/11) min past 7
(c) 5(3/11) min past 7
(d) 5(5/11) min past 7
Que 15: At what time between 5.30 and 6 will the hands of a clock be at right angles?
(a) 43(5/11) min past 5
(b) 43(7/11) min past 5
(c) 55 min past 5
(d) 47(5/11) min past 5
Answer Key:
1.(a), 2.(b), 3.(a), 4.(d), 5.(a), 6.(d), 7.(b), 8.(b), 9.(a), 10.(b), 11.(c), 12.(a), 13.(b), 14.(d), 15.(b)
Solution:
1. Case (i)⇒ \theta=(30H-\frac{11}{2}M).
90=(30\times 4-\frac{11}{2}M).
M=5\frac{5}{11} minute
Case (ii)⇒ \theta =(\frac{11}{2}M-30H).
90=(\frac{11}{2}M-30\times 4).
M=38\frac{2}{11} minute
Time Difference= 38\frac{2}{11}-5\frac{5}{11}=32\frac{8}{11} min
2. The minute hand crosses the hour hand for the sixth time exactly after every 6 hrs 36 min 30 sec of watch time. (Clock MCQ with Solutions)
It means, the time, taken to cross each time= 1 hr 6 min 5 sec= 1 hr 6\frac{1}{12} min
In a normal clock, the two hands meet at an interval of 65\frac{5}{11} minutes.
65\frac{5}{11}= 1 hr 5\frac{5}{11} min
The time taken by this clock is more than normal so the clock is losing time.
Time difference (Loss per meeting)= 1 hr 6\frac{1}{12} min – 1 hr 5\frac{5}{11} min =\frac{83}{132} min
In each 65\frac{5}{11} min clock loses ⇒\frac{83}{132} min
\frac{720}{11} min⇒\frac{83}{132} min
1 min⇒\frac{83}{132}\times \frac{11}{720} min
1 day= 24×60×1 min⇒\frac{83}{132}\times \frac{11}{720}\times 24\times 60 min
1 day⇒\frac{83}{6} min
1 day⇒13\frac{5}{6} min= 13 min 50 sec
So, 13 min 50 sec lost per day (Clock MCQ with Solutions)
3. Time difference from Sunday morning at 8 am to next Sunday at 8 pm= 7×24+12 =180 hours
The clock, gains⇒6 min+6 min 48 sec= \frac{64}{5} min
\frac{64}{5} min gain⇒ 180 hours
6 min gain⇒ 180\times \frac{5}{64}\times 6 =84\frac{3}{8} hr
84\frac{3}{8} hr= 3 days 12 hrs 22 min 30 sec
8 am Sunday +3 days 12 hrs 22 min 30 sec ⇒8:22:30 PM Wednesday (Clock MCQ with Solutions)
4. The clock loses 16 minutes in 24 hours.
It means \frac{16}{24}=\frac{2}{3} min or 40 seconds/hr
Clock loses time means it is slow.
1 hr – 40 sec in faulty clock⇒ 1 hr of normal clock
\frac{89}{90} hr⇒ 1 hr
1 hr⇒ \frac{90}{89} hr ……….(i)
The time interval between 5 am today to 10 pm of the 4th day= 3×24+17= 89 hours
From equation (i)
89 hours, of faulty clock⇒ \frac{90}{89}\times 89 hr =90 hours of Normal clock
So, the true time is 11 pm. (Clock MCQ with Solutions)
5. Hands meet every 64 minutes
Coinciding time< 65\frac{5}{11} min
So, the clock is going faster than normal (gaining time)
In 720/11 min, gain⇒ 65\frac{5}{11} -64= \frac{16}{11} min
In 1 min, gain⇒ \frac{1}{45} min
In 1 day, gain⇒ \frac{1}{45}\times 60\times 24 min= 32 min
6. \theta=(30H-\frac{11}{2}M).
H=10 and M=25
=162.5°
Reflex Angle= 360°-162.5°=197.5°
7. A clock that gains 5 seconds in 3 minutes is correct at 7 in the morning
7 am to 4:15 pm⇒ 9 hr 15 min
=9×60×60+ 15×60 Sec
=555×60 Sec (Wrong Time)
| Wrong | Right |
| 185 Sec | 180 Sec |
| 1 Sec | \frac{180}{185} Sec |
| 555×60 Sec | 555\times 60\times \frac{180}{185}=3\times 60\times 180 Sec |
3×60×180 Sec= 9×60×60 Sec= 9 hr
7 am +9 hr= 4 pm
8. 2 minutes slow on Monday at noon and 4 minutes 48 seconds fast on the next Monday at 2 pm.
12 pm Monday to 2 pm next Monday⇒ 7×24+2= 170 hr
2 min+ 4 min 48 sec= 408 sec
408 sec⇒ 170 hr
1 sec⇒ \frac{5}{12} hr
120 sec⇒ 50 hr (2 days and 2 hr)
12 pm Monday+ 50 hr⇒ 2 pm Wednesday (Clock MCQ with solutions)
9. 10 pm on 21st January 2010 to 4 pm on 22nd January 2010⇒ 18 hr
In 18 hr, The first clock gains= 18×2= 36 min
The second clock loses= 18×5= 90 min
Time difference= 36+90= 126 min (Clock MCQ with solutions)
10. A wall clock gains 2 minutes every 12 hours, while a table clock loses 2 minutes every 36 hours.
Wall Clock gains⇒ 4 min/day
Table clock loses⇒ 4/3 min/day
The time difference in 1 day= 4+4/3= 16/3 min
16/3 min⇒ 1 day
1 min⇒3/16 day
12 hrs⇒ \frac{3}{16}\times 12\times 60=135 days
Note: When this time interval becomes equal to 12 hours, both clocks will show the same time (regardless of am and pm). (Clock MCQ with solutions)
11. In 25 min, the minute hand gains, over the hour hand by:
The angle of rotation of the hour hand in 25 minutes =25×0.5° = 12.5°
The angle of rotation of the minute hand in 25 minutes =25×6° = 150°
Gain= 150°- 12.5°= 137.5°
12. A clock gains 15 min per day. (Clock MCQ with solutions)
12 noon to 4 am⇒ 16 hr
Gain in 16 hr= \frac{15}{24}\times 16 = 10 min
So, the right time will be 4:10 am
13. If a clock strikes 12 in 33 seconds, it will strike 6 in how many seconds
In order to strike 12 there are 11 intervals so the required number of intervals to strike 6 would be 5.
The required time= \frac{33}{11}\times 5= 15 sec
14. Between 7 and 8 O’clock will the hands of a clock be in the same straight line but not together?
The angle between the hands of the clock= 180°
\theta=(30H-\frac{11}{2}M).
H=7, 𝜃=180°
M=5\frac{5}{11} min
The required time= 5(5/11) min past 7 (Clock MCQ with solutions)
15. Between 5.30 and 6 will the hands of a clock be at right angles
\theta=(\frac{11}{2}M-30H).
H=5, 𝜃=90°
M=43\frac{7}{11} min
The required Time= 43(7/11) min past 5
Calendar problems with Solutions: Click Here
